Difference between revisions of "Category:Equilibrium constraints"
From mintOC
m (Initial setup of IMA paper text) |
m |
||
| (One intermediate revision by the same user not shown) | |||
| Line 4: | Line 4: | ||
<math> | <math> | ||
\begin{array}{llcl} | \begin{array}{llcl} | ||
| − | \displaystyle \min_{y_1, y_2, y_3} & \Phi(y_1, y_2, y_3) \\[1.5ex] | + | \displaystyle \min_{y_1, y_2, y_3} & & & \Phi(y_1, y_2, y_3) \\[1.5ex] |
\mbox{s.t.} & 0 & = & F ( y_1, y_2, y_3), \\ | \mbox{s.t.} & 0 & = & F ( y_1, y_2, y_3), \\ | ||
& 0 & \le & C ( y_1, y_2, y_3), \\ | & 0 & \le & C ( y_1, y_2, y_3), \\ | ||
| − | & 0 & \le & y_1 \ | + | & 0 & \le & (\mu - y_2)^T \; \phi( y_1, y_2), \; y_2 \in Y(y_1), \; \forall \mu \in Y(y_1), |
\end{array} | \end{array} | ||
</math> | </math> | ||
| Line 13: | Line 13: | ||
where <math>Y(y_1)</math> is the feasible region for the variational inequality and given function <math>\phi(\cdot)</math>. Variational inequalities arise in many domains and are generally referred to as equilibrium constraints. The variables <math>y_1</math> and <math>y_2</math> may be controls or states. | where <math>Y(y_1)</math> is the feasible region for the variational inequality and given function <math>\phi(\cdot)</math>. Variational inequalities arise in many domains and are generally referred to as equilibrium constraints. The variables <math>y_1</math> and <math>y_2</math> may be controls or states. | ||
| + | |||
| + | [[:Category:Complementarity constraints | Complementarity constraints]] and [[:Category:Vanishing constraints | vanishing constraints]] are special cases. | ||
[[Category:Objective characterization]] | [[Category:Objective characterization]] | ||
Latest revision as of 13:15, 20 November 2010
This category contains mathematical programs with equilibrium constraints (MPECs). An MPEC is an optimization problem constrained by a variational inequality, which takes for generic variables / functions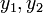 
the following general form:
![\begin{array}{llcl}
\displaystyle \min_{y_1, y_2, y_3} & & & \Phi(y_1, y_2, y_3) \\[1.5ex]
\mbox{s.t.} & 0 & = & F ( y_1, y_2, y_3), \\
& 0 & \le & C ( y_1, y_2, y_3), \\
& 0 & \le & (\mu - y_2)^T \; \phi( y_1, y_2), \; y_2 \in Y(y_1), \; \forall \mu \in Y(y_1),
\end{array}](https://mintoc.de/images/math/f/4/d/f4d20f04f516a6ae8307a4f50497aed6.png)
where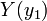 
is the feasible region for the variational inequality and given function 
. Variational inequalities arise in many domains and are generally referred to as equilibrium constraints. The variables 
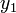and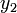 
may be controls or states.
Complementarity constraints and vanishing constraints are special cases.
This category currently contains no pages or media.
