Difference between revisions of "Diels-Alder Reaction Experimental Design"
From mintOC
(→Model Formulation) |
(→Model Formulation) |
||
| Line 40: | Line 40: | ||
\displaystyle \min_{x, G, F, u} & trace(F^{-1} (t_{t_f})) \\[1.5ex] | \displaystyle \min_{x, G, F, u} & trace(F^{-1} (t_{t_f})) \\[1.5ex] | ||
\mbox{s.t.} & \dot{x} = f(x,u,p,t), \forall \, t \in I\\ | \mbox{s.t.} & \dot{x} = f(x,u,p,t), \forall \, t \in I\\ | ||
| − | & \dot{n_1}(t) = -k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\ | + | & \dot{n_1}(t) = -k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\ |
| − | + | & \dot{n_2}(t) = -k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\ | |
| − | & \dot{n_2}(t) = -k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\ | + | & \dot{n_2}(t) = \ \ k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\ |
| − | + | ||
| − | & \dot{n_2}(t) = \ \ k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}} | + | |
& 0 = g(x(t_o),x(t_f),p) \\ | & 0 = g(x(t_o),x(t_f),p) \\ | ||
& 0 \ge c(x,u,p), \forall \, t \in I\\ | & 0 \ge c(x,u,p), \forall \, t \in I\\ | ||
Revision as of 12:27, 4 December 2015
This page can now be filled with content.
Model Formulation
Differential equation system:
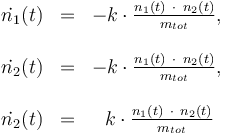
Reaction velocity constant:

Total mass:
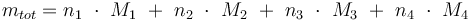
Temperature in Kelvin:
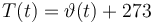
![\begin{array}{cl}
\displaystyle \min_{x, G, F, u} & trace(F^{-1} (t_{t_f})) \\[1.5ex]
\mbox{s.t.} & \dot{x} = f(x,u,p,t), \forall \, t \in I\\
& \dot{n_1}(t) = -k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\
& \dot{n_2}(t) = -k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\
& \dot{n_2}(t) = \ \ k \cdot \frac{n_1(t) \ \cdot \ n_2(t)}{m_{tot}}, \\
& 0 = g(x(t_o),x(t_f),p) \\
& 0 \ge c(x,u,p), \forall \, t \in I\\
& 0 = h(x,u,p), \forall \, t \in I \\
& x \in \mathcal{X},\,u \in \mathcal{U},\, p \in P.
\end{array}](https://mintoc.de/images/math/8/9/f/89fb02dedc5b0345a139f34f746e60b0.png)
| Name | Symbol | Initial value ( ) )
|
| Molar number 1 | 
|
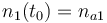
|
| Molar number 2 | 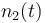
|
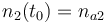
|
| Molar number 3 | 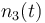
|
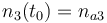
|
| Name | Symbol | Value |
| Molar Mass | 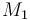
|
0.1362 |
| Molar Mass | 
|
0.09806 |
| Molar Mass | 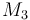
|
0.23426 |
| Molar Mass | 
|
0.236 |
| Universal gas constant | 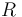
|
8.314 |
| Reference temperature | 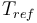
|
293 |
| Name | Symbol | Value |
| Steric factor | 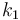
|
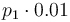
|
| Steric factor | 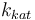
|
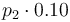
|
| Activation energie | 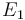
|
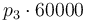
|
| Activation energie | 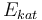
|
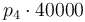
|
| Catalyst deactivation coefficient | 
|
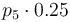
|
with 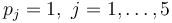
| Name | Symbol | Interval |
| Initial molar number 1 | 
|
[0.4,9.0] |
| Initial molar number 2 | 
|
[0.4,9.0] |
| Initial molar number 3 | 
|
[0.4,9.0] |
| Concentration of the catalyst | 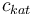
|
[0.0,6.0] |
| Initial molar number 1 | 
|
[20.0,100.0] |