Difference between revisions of "Annihilation of calcium oscillations with PLC activation inhibition"

From mintOC
Jump to: navigation, search
m (Text replacement - "<bibreferences/>" to "<biblist />")
m (Text replacement - "\<bibref\>(.*)\<\/bibref\>" to "<bib id="$1" />")
Line 32: Line 32:
 
== Reference Solutions ==
 
== Reference Solutions ==
  
−
A solution for this problem is described in <bibref>Lebiedz2005</bibref>. A local minimum that is actually slightly worse than the solution provided for only one control, is shown in the next plots.
+
A solution for this problem is described in <bib id="Lebiedz2005" />. A local minimum that is actually slightly worse than the solution provided for only one control, is shown in the next plots.
  
 
<gallery caption="Reference solution plots" widths="180px" heights="140px" perrow="2">
 
<gallery caption="Reference solution plots" widths="180px" heights="140px" perrow="2">

Revision as of 21:30, 30 December 2015

Annihilation of calcium oscillations with PLC activation inhibition
State dimension: 1
Differential states: 4
Discrete control functions: 2
Interior point equalities: 4


This control problem is closely related to Annihilation of calcium oscillations. The only difference is an additional control function, the inhibition of PLC activation. We state only the differences in this article.

Mathematical formulation

For t \in [t_0, t_f] almost everywhere the mixed-integer optimal control problem is given by

\begin{array}{llcl} \displaystyle \min_{x, w, w^{\mathrm{max}}} & & & {\int_{t_0}^{t_f} || x(\tau) - \tilde{x} ||_2^2 + p_1 w_1(\tau) + p_2 w_2(\tau) \; \mathrm{d}\tau} \\[1.5ex] \mbox{s.t.} & \dot{x}_0 & = & k_1 + k_2 x_0 - \frac{k_3 x_0 x_1}{x_0 + K_4} - \frac{k_5 x_0 x_2}{x_0 + K_6} \\ & \dot{x}_1 & = & (1 - w_2) k_7 x_0 - \frac{k_8 x_1}{x_1 + K_9} \\ & \dot{x}_2 & = & \frac{k_{10} x_1 x_2 x_3}{x_3 + K_{11}} + k_{12} x_1 + k_{13} x_0 - \frac{k_{14} x_2}{w_1 \cdot x_2 + K_{15}} - \frac{k_{16} x_2}{x_2 + K_{17}} + \frac{x_3}{10} \\ & \dot{x}_3 & = & - \frac{k_{10} x_1 x_2 x_3}{x_3 + K_{11}} + \frac{k_{16} x_2}{x_2 + K_{17}} - \frac{x_3}{10} \\[1.5ex] & x(0) &=& (0.03966, 1.09799, 0.00142, 1.65431)^T, \\ & x(t) & \ge & 0.0, \\ & w_1(t) &\in& \{1, w^{\mathrm{max}}\}, \\ & w_2(t) &\in& \{0, 1\}, \\ & w^{\mathrm{max}} & \ge & 1.1, \\ & w^{\mathrm{max}} & \le & 1.3. \end{array}

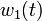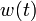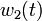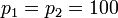Note that we write w_1(t) instead of w(t) and have an additional control function w_2(t). The regularization parameters are set to p_1 = p_2 = 100.

Reference Solutions

A solution for this problem is described in [Lebiedz2005]Author: Lebiedz, D.; Sager, S.; Bock, H.G.; Lebiedz, P.
Journal: Physical Review Letters
Pages: 108303
Title: Annihilation of limit cycle oscillations by identification of critical phase resetting stimuli via mixed-integer optimal control methods
Volume: 95
Year: 2005
Link to Google Scholar. A local minimum that is actually slightly worse than the solution provided for only one control, is shown in the next plots.

  • Reference solution plots

  • Optimal stimuli determined by mixed-integer optimal control.

  • Corresponding differential states.

Variants

Only one control function.

References

[Lebiedz2005]Lebiedz, D.; Sager, S.; Bock, H.G.; Lebiedz, P. (2005): Annihilation of limit cycle oscillations by identification of critical phase resetting stimuli via mixed-integer optimal control methods. Physical Review Letters, 95, 108303Link to Google Scholar
Retrieved from "https://mintoc.de/index.php?title=Annihilation_of_calcium_oscillations_with_PLC_activation_inhibition&oldid=1106"