Difference between revisions of "Control of Heat Equation with Actuator Placement"
FelixMueller (Talk | contribs) |
FelixMueller (Talk | contribs) |
||
| Line 4: | Line 4: | ||
|nu = <math>L</math> | |nu = <math>L</math> | ||
|nw = <math>L</math> | |nw = <math>L</math> | ||
| − | |nre = | + | |nc = 3 |
| + | |nre = 2 | ||
}}<!-- Do not insert line break here or Dimensions Box moves up in the layout... | }}<!-- Do not insert line break here or Dimensions Box moves up in the layout... | ||
Revision as of 16:16, 23 February 2016
| Control of Heat Equation with Actuator Placement | |
|---|---|
| State dimension: | 1 |
| Differential states: | 1 |
| Continuous control functions: |
 |
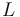| Discrete control functions: |
|
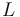| Path constraints: | 3 |
| Interior point equalities: | 2 |
This problem is governed by the heat equation and is adapted from Iftime and Demetriou ([Iftime2009]Author: Orest V. Iftime; Michael A. Demetriou
Journal: {A}utomatica
Number: 2
Pages: 312--323
Title: {O}ptimal control of switched distributed parameter systems with spatially scheduled actuators
Volume: 45
Year: 2009
).
Its goal is to choose a place to apply an actuator in a given area depending on time.
We consider a rectangle ![\Omega=[0,1]\times[0,2]](https://mintoc.de/images/math/b/9/e/b9e9ecbd1ca99669f6d13a62bcf6142b.png)
with the boundary 
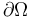and the time horizon ![T=[0,10]](https://mintoc.de/images/math/0/a/e/0aef63dc3199780b7093eeb4bd8d74f5.png)
as the domains.
The objective function is quadratic, its first term captures the desired final state 
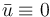, the second term regularize the state over time and the third term regularize the continuous controls.
The constraints are a source budget, which limits the quantity of placed actuators, and the two-dimensional heat equation with some source function.
Additionally, we assume Dirichlet boundary conditions and initial conditions.
Mathematical formulation
Parameters
Reference solution
Source Code
References
| [Iftime2009] | Orest V. Iftime; Michael A. Demetriou (2009): {O}ptimal control of switched distributed parameter systems with spatially scheduled actuators . {A}utomatica, 45, 312--323 | |
