Difference between revisions of "Category:Hyperbolic"
From mintOC
FelixMueller (Talk | contribs) |
FelixMueller (Talk | contribs) |
||
| Line 1: | Line 1: | ||
This category contains all control problems which are governed by a hyperbolic partial differential equation. | This category contains all control problems which are governed by a hyperbolic partial differential equation. | ||
| − | + | ||
| + | <p> | ||
A second order linear partial differential equation can be written as | A second order linear partial differential equation can be written as | ||
| − | <math>\sum^n_{i,j=1} a_{ij} \frac{\partial^2u}{\partial x_i \partial x_j} +\ | + | <math>\sum^n_{i,j=1} a_{ij} \frac{\partial^2u}{\partial x_i \partial x_j} +\, \text{lower-order terms} = 0</math>. |
| − | + | </p> | |
| − | If <math>A=(a_{ij})_{ij}</math> is indefinite such that <math>n-1</math> eigenvalues have the same sign and the remaining eigenvalue the other sign, the partial differential equation is called hyperbolic. | + | |
| − | + | <p> | |
| + | If the matrix <math>A=(a_{ij})_{ij}</math> is indefinite such that <math>n-1</math> eigenvalues have the same sign and the remaining eigenvalue has the other sign, the partial differential equation is called hyperbolic. | ||
| + | </p> | ||
| + | <p> | ||
An example is the wave equation: <math>\frac{\partial^2 u}{\partial t^2}-\Delta u = f</math>, | An example is the wave equation: <math>\frac{\partial^2 u}{\partial t^2}-\Delta u = f</math>, | ||
where <math>\Delta</math> denotes the Laplace operator, <math>u</math> is the unknown, and the function <math>f</math> is given. | where <math>\Delta</math> denotes the Laplace operator, <math>u</math> is the unknown, and the function <math>f</math> is given. | ||
| − | </p> | + | </p> |
<!--List of all categories this page is part of. List characterization of solution behavior, model properties, ore presence of implementation details (e.g., AMPL for AMPL model) here --> | <!--List of all categories this page is part of. List characterization of solution behavior, model properties, ore presence of implementation details (e.g., AMPL for AMPL model) here --> | ||
Latest revision as of 16:24, 24 February 2016
This category contains all control problems which are governed by a hyperbolic partial differential equation.
A second order linear partial differential equation can be written as
.
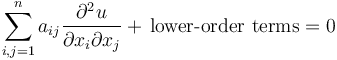If the matrix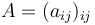 
is indefinite such that 
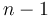eigenvalues have the same sign and the remaining eigenvalue has the other sign, the partial differential equation is called hyperbolic.
An example is the wave equation: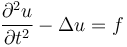 
,
where 
denotes the Laplace operator,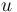 
is the unknown, and the function 
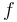is given.
Pages in category "Hyperbolic"
This category contains only the following page.
