Difference between revisions of "Lotka Volterra multi-arcs problem"

From mintOC
Jump to: navigation, search
(Created page with "{{Dimensions |nd = 1 |nx = 3 |nw = 1 |nre = 3 }}<!-- Do not insert line break here or Dimensions Box moves up in the layout... -->This site describ...")
 
Line 36: Line 36:
 
[t_0, t_f] &=& [0, 18],\\
 
[t_0, t_f] &=& [0, 18],\\
 
(c_0, c_1,) &=& (0.4, 0.2),\\
 
(c_0, c_1,) &=& (0.4, 0.2),\\
−
p_{0}(t) &=& \left\{\begin{array}[ll]1 & \text{for } t\in[0, 7.2] \end{array} \right. \\
+
p_{0}(t) &=& \left\{ \begin{array}{ll}
 +
1 \quad \quad &\text{for } \ t\in [0,7.2],\\
 +
0.9 \quad \quad &\text{for } \ t\in [7.2,12],\\
 +
1 \quad \quad &\text{for } \ t\in [12,18].
 +
\end{array} \right. \\
 
p_1(t) &=& p_0(t).
 
p_1(t) &=& p_0(t).
 
\end{array}
 
\end{array}
Line 45: Line 49:
 
If the problem is relaxed, i.e., we demand that <math>w(t)</math> be in the continuous interval <math>[0, 1]</math> instead of the binary choice <math>\{0,1\}</math>, the optimal solution can be determined by means of direct optimal control.  
 
If the problem is relaxed, i.e., we demand that <math>w(t)</math> be in the continuous interval <math>[0, 1]</math> instead of the binary choice <math>\{0,1\}</math>, the optimal solution can be determined by means of direct optimal control.  
  
−
The optimal objective value of the relaxed problem with  <math> n_t=12000, \, n_u=400 </math> is <math>x_2(t_f) =1.82875272</math>. The objective value of the binary controls obtained by Combinatorial Integral Approimation (CIA) is <math>x_2(t_f) =1.82878681</math>.   
+
The optimal objective value of the relaxed problem with  <math> n_t=18000, \, n_u=300 </math> is <math>x_2(t_f) =1.41842699</math>. The objective value of the binary controls obtained by Combinatorial Integral Approimation (CIA) is <math>x_2(t_f) =1.4237735 </math>.   
  
 
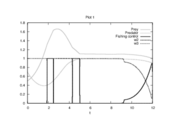
<gallery caption="Reference solution plots" widths="180px" heights="140px" perrow="2">
 
<gallery caption="Reference solution plots" widths="180px" heights="140px" perrow="2">
−
  Image:MmlotkaRelaxed_12000_30_1.png| Optimal relaxed controls and states determined by an direct approach with ampl_mintoc (Radau collocation)  and <math>n_t=12000, \, n_u=400</math>.
+
  Image:MmlotkaRelaxed_12000_30_1.png| Optimal relaxed controls and states determined by an direct approach with ampl_mintoc (Radau collocation)  and <math>n_t=18000, \, n_u=300</math>.
−
  Image:MmlotkaCIA 12000 30 1.png| Optimal binary controls and states determined by an direct approach (Radau collocation) with ampl_mintoc and <math>n_t=12000, \, n_u=400</math>. The relaxed controls were approximated by Combinatorial Integral Approximation.
+
  Image:MmlotkaCIA 12000 30 1.png| Optimal binary controls and states determined by an direct approach (Radau collocation) with ampl_mintoc and <math>n_t=18000, \, n_u=300</math>. The relaxed controls were approximated by Combinatorial Integral Approximation.
 
</gallery>
 
</gallery>
  

Revision as of 14:10, 21 December 2017

Lotka Volterra multi-arcs problem
State dimension: 1
Differential states: 3
Discrete control functions: 1
Interior point equalities: 3

This site describes a Lotka Volterra variant with three singular arcs instead of only one in the standard variant.

Mathematical formulation

The mixed-integer optimal control problem is given by

\begin{array}{llclr} \displaystyle \min_{x, w} & x_2(t_f) \\[1.5ex] \mbox{s.t.} & \dot{x}_0 & = & x_0 - x_0 x_1 - \; c_0\; x_0 \; w, \\ & \dot{x}_1 & = & - x_1 + x_0 x_1 - \; c_1\; x_1 \; w, \\ & \dot{x}_2 & = & (x_0 - p_0(t))^2 + (x_1 - p_1(t))^2, \\[1.5ex] & x(0) &=& (0.5, 0.7, 0)^T, \\ & w(t) &\in& \{0, 1\}, \end{array}

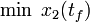Here the differential states (x_0, x_1) describe the biomasses of prey and predator, respectively. The third differential state is used here to transform the objective, an integrated deviation, into the Mayer formulation \min \; x_2(t_f). This problem variant allows to choose between three different fishing options.

Parameters

These fixed values are used within the model.

\begin{array}{rcl} [t_0, t_f] &=& [0, 18],\\ (c_0, c_1,) &=& (0.4, 0.2),\\ p_{0}(t) &=& \left\{ \begin{array}{ll} 1 \quad \quad &\text{for } \ t\in [0,7.2],\\ 0.9 \quad \quad &\text{for } \ t\in [7.2,12],\\ 1 \quad \quad &\text{for } \ t\in [12,18]. \end{array} \right. \\ p_1(t) &=& p_0(t). \end{array}

Reference Solutions

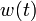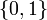If the problem is relaxed, i.e., we demand that w(t) be in the continuous interval [0, 1] instead of the binary choice \{0,1\}, the optimal solution can be determined by means of direct optimal control.

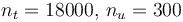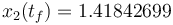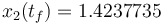The optimal objective value of the relaxed problem with n_t=18000, \, n_u=300 is x_2(t_f) =1.41842699. The objective value of the binary controls obtained by Combinatorial Integral Approimation (CIA) is x_2(t_f) =1.4237735 .

  • Reference solution plots

  • Optimal relaxed controls and states determined by an direct approach with ampl_mintoc (Radau collocation) and n_t=18000, \, n_u=300.

  • Optimal binary controls and states determined by an direct approach (Radau collocation) with ampl_mintoc and n_t=18000, \, n_u=300. The relaxed controls were approximated by Combinatorial Integral Approximation.

Retrieved from "https://mintoc.de/index.php?title=Lotka_Volterra_multi-arcs_problem&oldid=2165"