Category:Vanishing constraints

From mintOC
Revision as of 11:10, 23 January 2016 by JonasSchulze (Talk | contribs) (Text replacement - "<bibreferences/>" to "<biblist />")

(diff) ← Older revision | Latest revision (diff) | Newer revision → (diff)
Jump to: navigation, search

This category contains mathematical programs with vanishing constraints (MPVCs). The problem

\begin{array}{llcl} \displaystyle \min_{y} & & & \Phi(y) \\[1.5ex] \mbox{s.t.} & 0 & \ge & g_i(y) h_i(y), \quad i \in \{1, \dots, m\}\\ & 0 & \le & \le h(y) \\ \end{array}

with smooth functions 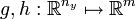g, h: \R^{n_y} \mapsto \R^m is called MPVC. Note that every MPVC can be transformed into an MPEC [Achtziger2008]Author: W. Achtziger; C. Kanzow
Journal: Mathematical Programming Series A
Pages: 69--99
Title: Mathematical programs with vanishing constraints: optimality conditions and constraint qualifications
Volume: 114
Year: 2008
Link to Google Scholar[Izmailov2009]Author: A.F. Izmailov; M.V. Solodov
Journal: Journal of Optimization Theory and Applications
Pages: 501--532
Title: Mathematical Programs with Vanishing Constraints: Optimality Conditions, Sensitivity, and a Relaxation Method
Volume: 142
Year: 2009
Link to Google Scholar. Examples for vanishing constraints are engine speed constraints that are only active if the corresponding gear control is nonzero.

References

[Achtziger2008]W. Achtziger; C. Kanzow (2008): Mathematical programs with vanishing constraints: optimality conditions and constraint qualifications. Mathematical Programming Series A, 114, 69--99Link to Google Scholar
[Izmailov2009]A.F. Izmailov; M.V. Solodov (2009): Mathematical Programs with Vanishing Constraints: Optimality Conditions, Sensitivity, and a Relaxation Method. Journal of Optimization Theory and Applications, 142, 501--532Link to Google Scholar

Pages in category "Vanishing constraints"

This category contains only the following page.

C

Retrieved from "https://mintoc.de/index.php?title=Category:Vanishing_constraints&oldid=1384"