Difference between revisions of "Category:Solution characterization"
JonasSchulze (Talk | contribs) m (Text replacement - "\<bibref\>(.*)\<\/bibref\>" to "<bib id="$1" />") |
FelixMueller (Talk | contribs) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 4: | Line 4: | ||
== References == | == References == | ||
| − | < | + | <biblist /> |
| + | |||
[[Category:Problem characterization]] | [[Category:Problem characterization]] | ||
Latest revision as of 13:58, 28 January 2016
The classification that we propose for switching decisions is based on insight from Pontryagin's maximum principle [Pontryagin1962]Address: Chichester
Author: Pontryagin, L.S.; Boltyanski, V.G.; Gamkrelidze, R.V.; Miscenko, E.F.
Publisher: Wiley
Title: The Mathematical Theory of Optimal Processes
Year: 1962
applied here only to the relaxation of the binary control functions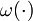 
, denoted by ![\alpha(\cdot) \in [0,1]^{n_\omega}](https://mintoc.de/images/math/6/d/4/6d44af77747bea2951d669a09e98b55d.png)
. In the analysis of linear control problems one distinguishes three cases: bang-bang arcs, sensitivity-seeking arcs, and path-constrained arcs, [Srinivasan2003]Author: Srinivasan, B.; Palanki, S.; Bonvin, D.
Journal: Computers \& Chemical Engineering
Pages: 1--26
Title: Dynamic Optimization of Batch Processes: I. Characterization of the Nominal Solution
Volume: 27
Year: 2003, where an arc is defined to be a nonzero time-interval. Of course a problem's solution can show two or even all three behaviors at once on different time arcs.
Additionally we characterize solutions, whenever chattering or sliding mode behavior occurs.
References
| [Pontryagin1962] | Pontryagin, L.S.; Boltyanski, V.G.; Gamkrelidze, R.V.; Miscenko, E.F. (1962): The Mathematical Theory of Optimal Processes. (%edition%). Wiley, Chichester, %pages% | |
| [Srinivasan2003] | Srinivasan, B.; Palanki, S.; Bonvin, D. (2003): Dynamic Optimization of Batch Processes: I. Characterization of the Nominal Solution. Computers \& Chemical Engineering, 27, 1--26 | |
Subcategories
This category has the following 5 subcategories, out of 5 total.
