Oil Shale Pyrolysis

Oil Shale Pyrolysis
State dimension:	1
Differential states:	2
Continuous control functions:	1
Discrete control functions:	0
Interior point equalities:	2

The following problem is an example from the global optimal control literature and was introduced in <bibref>Wen1977</bibref>.

Mathematical formulation

$\begin{array}{ll} \displaystyle \min_{u} & \displaystyle -x_1(t_N)^2 \\[1.5ex] \mbox{s.t.} & \displaystyle \dot{x}_0(t) = -k_0x_0(t)-(k_2+k_3+k_4)x_0(t)x_1(t)\\ & \displaystyle \dot{x}_1(t) = k_0x_0(t)-k_1x_1(t) + k_2x_0(t)x_1(t)\\ & \displaystyle k_i = a_i e^{-u(t)\frac{b_i}{R}},\quad \forall i\in \{1,\dots,5\} \\ [1.5ex] & \displaystyle t \in \left[t_0,t_N\right] \\ & \displaystyle u(t) \in \left[698.15/748.15,1\right]\\ & \displaystyle x(t_0) = (1,0)^T\\ \end{array}$

where this is the normalized form with

$u(t)= \frac{1}{u_{temp}}$, with

$u_{temp} \in \left[698.15,748.15\right]$

Parameters

State variables

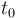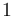
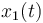
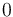
Symbol	Initial value ($t_0$)
$x_0(t)$	$1$
$x_1(t)$	$0$

Parameters

Symbol	Value
$a_1$	$8.86$
$a_2$	$24.25$
$a_3$	$23.67$
$a_4$	$18.75$
$a_5$	$20.7$
$b_1$	$20.3$
$b_2$	$37.4$
$b_3$	$33.8$
$b_4$	$28.2$
$b_5$	$31.0$

Control variable

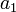
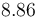
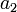
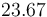
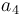
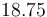
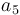
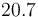
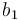
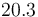
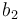
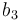
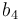
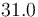
Symbol	Interval
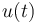
$u(t)$	[698.15/748.15,1]

Measurement grid

Reference solution

Coming soon.

References

There were no citations found in the article.